• Refractive power is measured in dioptres (D)

• Power = 1/focal length of lens (m)

• The total refractive power of the eye is around 60D

  • Cornea = 43D
  • Lens = 17D
    

    

• An eye which has a higher refractive power than normal will be myopic

  • As power increases, focal length decreases and the image is focused at a shorter distance
  • (in front of the retina)
  • Myopes are short-sighted because a higher-powered eye focuses the light in front of the retina, rather than on it.
  • When an object of visual focus is brought nearer, the person sees it clearer. This is because moving the object closer pushes the point of focus further back and onto the retina.

• An eye which has a lower refractive power than normal will be hyperopic

  • As power decreases, focal length increases and the image is focused at a longer distance (behind the retina)
  • Hyperopes are long-sighted because a lower-powered eye focuses the light behind the retina, rather than on it
  • When an object of visual focus is moved further away, the person sees it clearer. This is because moving the object further, pulls the point of focus from behind the retina.

• Astigmatism is when the refractive power is not uniform over a structure i.e if the left side of the corneal surface is steeper (higher power) than the right

  • In astigmatism, light is not accurately focused, so the patient experiences blurry vision

The visible spectrum of light is 380nm-750nm, in the typical human eye.

• Axial length of the eye is the distance between the tear film and the retinal pigment epithelium
• The average axial length is around 23.5mm, the normal range is 22-24mm
• Axial length can be measured using interferometry
• An eye which is longer is myopic, the light will be focused in front of the retina
  • Myopes are short-sighted
  • When the object of visual focus is brought nearer, the person sees it clearer. This is because the point of focus is moved backwards and onto the retina
• An eye which is shorter is hyperopic, the point of focus will be behind the retina
  • Hyperopes are long-sighted
  • When the object of visual focus is moved further, the person sees it clearer. This is because the point of focus is moved forwards and onto the retina.
• Axial length does not have a significant impact on astigmatism

Spherical

• Used to correct ametropia and presbyopia
• -ve D lenses are concave and diverge light → correct myopia
  • A myopic patient
• +ve D lenses are convex and converge light → correct hyperopia and presbyopia
  • E.g : A hyperopic patient would like spectacles to allow reading at 20cm. What power should the lenses be?
    • The lens has to have a focal point which matches the far point
    • The far point in this case is 20cm, so a lens with a focal length of 20cm is needed
    • Power=1/focal length(m)
    • P=1/0.2 = 5
    • Answer = +5D

Toric

• Used to correct astigmatism
• Is composed of a sphere and cylinder
  • The sphere acts in all meridians
  • The cylinder only acts in a specific meridian, this allows it to treat astigmatism.

These principles apply to all kinds of lenses, from contacts to spectacles and IOLs

LVC focuses on moulding the corneal surface. You can make it steeper to increase power or flatter to decrease it.

LASIK

• Laser assisted in-situ keratomileusis
• A flap is created on the corneal epithelium and an excimer laser is used to mould the corneal stroma. The flap is then repositioned

LASEK

• Laser assisted subepithelial keratomileusis
• Alcohol is spread on the corneal epithelium, this loses it and allows it to the moved to expose the stroma. An excimer laser is used to mould the corneal stroma. The epithelium is then repositioned

PRK

• Photorefractive keratectomy
• The same process as LASEK but the epithelium is discarded rather than repositioned. A bandage contact lens is used for healing

SMILE

• Small incision lenticule extraction
• Uses a femtosecond laser to remove a lens-shaped disc of the corneal stroma

• You can clip an artificial lens in front of the human crystalline lens as an alternative to LVC or where LVC is contraindicated
• Visian lens is implanted onto the ciliary sulcus
• Artisan lens is clipped onto the anterior iris
• Pseudophakic lens procedures are when the human crystalline lens is removed altogether and replaced with an IOL implant.

• Physiological myopia is common and mild
• Pathological myopia is rare and characterised by continuous lengthening of the posterior segment and results in high myopia.
• Degenerative myopia is a distinct pathological myopia which is discussed in the retina chapter

• The severity of myopia is classified according to the strength of the lens that is needed to correct it. I.e a patient might have a total refraction of 63D, and will need -3D of correction. This patient's myopia is classified as -3D.
• <-3D = low myopia

• -6D = high myopia

• -ve D spherical lenses are used to correct myopia
• They are concave and diverge light

Mathematicians might argue that >-6 includes the numbers which are numerically greater than -6 (-5,-4,-3….). In this context however, greater than -6D of myopia refers to more negative numbers (-7,-8…).

Lens are prescribed in power(D). You can calculate the power of the lens required to correct myopia using the far point (the furthest distance a person can see clearly).

Example

• A patient can only see clearly up to 50 cm in the distance. Any further and his vision becomes blurry. What lens power is required to correct his short-sightedness?
  • The far point is 50cm = 0.5m
  • For our calculations you can simply equate the far point with the focal point and use the lens equation to calculate the power
    • The equation underlying this is the lens equation : 1/f = (1/I) + (1/O)
      • I = image = far point = -0.5
        • The negative value comes from the fact that this image is in front of the lens and is virtual
      • O = object distance = infinity
        • Theoretically, you want the patient to see to infinity → 1/00
          • For this reason, you can simply remove the 1/I component of the equation which leaves: 1/f=1/O → f=O
    • f = -0.5m
  • Lens power is P=1/f(m)
  • P = 1/-0.5 = -2D
  • Answer = -2D

• The severity of hyperopia is classified according to the strength of the lens that is needed to correct it.
• <+2D → low

• +5D → high

• +ve D spherical lenses are used to correct myopia
• These lenses are convex and they converge light

Lens are prescribed in power(D). You can calculate the power of the lens required to correct hyperopia using the near point (the closest a person can see clearly) and the target working distance

Examples

• A long-sighted person cannot see objects which are closer than 50cm. She wants a lens which will enable her to read at 25cm. What power of lens should be prescribed?
  • This question requires you to use the lens equation: 1/f=(1/O) + (1/I)
  • I = image distance = near point = 50cm
    - The image is in front of the lens, not on the retina. Therefore, it is virtual and attributes a negative value = -50cm
  • O = object distance = target point = 25cm
  • f = focal length.
    - Once found, f can be plugged into p=1/f(m) to calculate power
  • 1/f= (1/25) - (1/50) = 1/50cm
  • f= 50cm = 0.5m
  • 1/f(m)=P
  • 1/0.5 = +2D

Classification is important because informs management

Regular

• Regular astigmatism is when the astigmatic meridians are 90° apart
• It is subclassified as with-the-rule (WTR), against-the-rule (ATR) and oblique
  • WTR - steep meridian (higher power) is at 90°
  • ATR - steep meridian (higher power) is at 180°
  • Oblique is when the meridians are >20° away from 90° and 180°

Irregular

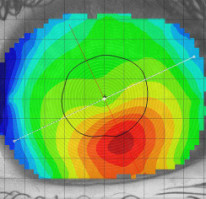
• Irregular astigmatism is most often due to corneal surface disorders such as scarring and keratoconus (discussed further in the cornea chapter)
• Irregular astigmatism is when the astigmatic meridians are irregular and not 90° apart

• Regular astigmatism can be treated with soft toric lenses
  • Toric lenses use a cylinder which focuses light on a particular meridian
  • This cylinder can be nested within a spherical lens, where the sphere affects all the meridians equally and the cylinder focuses on a particular meridian
• Irregular astigmatism is treated with rigid gas permeable contact lenses (RGP CLs)

Transposition is a way of altering a lens prescription of a cylinder where a minus lens is changed to a plus, or vice versa

• A toric lens prescription might look like this: +5DS/-2DC at 90°
  • DS → power of the spherical lens
  • DC → power of the cylindrical lens
  • 90° → the meridian that is perpendicular to the action of the cylinder. So there is no cylinder power at 90° and there is +2DC cylinder power at 180°. Relatively, there is -2DC at 90°
• Transposition of the above prescription would result in: +3Ds/+2DC at 180°
  • Calculation steps:
    • DS + DC → this value is your new DS (+3DS)
    • Change DC sign → (+2DC)
    • Change the axis
      • If original axis is <90° → add 90° (90° +90° = 180°)
      • If original axis is >90° → subtract 90°

The transposed prescription is equal to the original. The reason it is examined is because it tests your understanding of toric lens configuration. This can be a little complex to understand at first and if you’re struggling or short on time, simply follow the steps above and you will get the right answer every time.

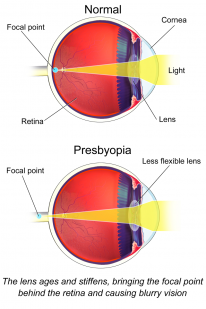
• With age, the lens hardens and the anterior capsule thickens
• Lens power decreases because it loses the ability to change shape with accommodation
• The decline of accommodative power with age:
  • At birth → 16D
  • 25 years → 8D
  • 50 years → 2D
  • 60 years → <1D
• People around the age of 50 invariably require reading glasses
• Presbyopia is delayed in myopes (short-sighted people)
• It is clinically defined as the inability to read binocularly (6/12 VA) at 40cm or at their habitual working distance, in a person >35yrs

There is currently no effective prevention for presbyopia.